It is perhaps unfortunate that the most common test for stability is to look for 'ringing' on a square-wave test signal. It is instructive to look at some examples, here using a 2kHz square wave input.
The first looks like sustained low level oscillation around 30kHz, while the second looks like damped oscillation at the same frequency. Actually the first diagram has nothing at all added to the square wave, the only thing done was to remove everything above the 15th harmonic. Everything up to and including 30kHz is being reproduced with no distortion, no phase error and flat frequency response. (If possible see 'A check on Fourier' by M.G.Scroggie, Wireless World, Nov 1977. p79-82. His Fig.5 is a better drawn version showing the harmonics and how they add.) The lack of higher frequency components however gives the impression of a serious problem, when in fact the audio frequency reproduction is perfect, and there is nothing at all added or removed in this range. The symmetrical variation of the 'oscillation' amplitude gives a clue to the origin of the effect, but practical low pass filters give a less sharp cut off of high harmonics together with frequency dependant phase shift which will give a different appearance. The suggestion that 'ringing' needs to be minimised is not entirely convincing when even an ideal low-pass filter gives the above result. Using an audio signal with no frequency components above 30kHz instead of the square wave there would be no effect at all from this filter.
The second diagram can also be the result of low-pass filtering, and something similar is often produced by the interaction of output inductors with capacitive loads, which is not related in any direct way to stability. Checking the signal ahead of the inductor may reveal a smooth signal without the 'ringing' effect, though some amplifiers have an output impedance with a small internal inductive component which will add some small effect. The square-wave response shown in the MJR-6 test results shows low level 'ringing' which is estimated at 120kHz. This is close to the expected resonance frequency of the 0.4uH output inductor with the 4uF load capacitance used in that test. Increasing loop gain to the point where the amplifier becomes unstable caused oscillation around 6MHz, as expected from the feedback loop unity gain frequency. This demonstrates that output 'ringing' is generally not related to instability, which can occur in an entirely different frequency range, and unless the input signal includes components close to the LC resonance frequency, or the inductance used is too high, there will be little effect. Leaving out the output inductor to eliminate 'ringing' caused by this LC resonance may seriously reduce the phase margin at higher frequencies with some capacitive loads, dangerously increasing the risk of instability.
A square wave test to investigate stability into capacitive loads is therefore of limited usefulness, and may be seriously misleading. My experience is that amplifiers sometimes have a stable state and an unstable state, and triggering them into instability may need a precise choice of load and input signal, in one case driving the amplifier heavily into clipping and then removing the input signal caused a dramatic latch-up and oscillation effect. Failure to oscillate with just any square-wave input and the usual 2uF test load may be necessary, but is no guarantee of unconditional stability. I also use high level sinewave signals at various frequencies, and look for signs of instability close to clipping as the signal level is adjusted to give different levels of clipping. Going into or out of clipping the loop gain is changing, and so the feedback loop unity gain frequency is in effect shifted over a wide range, revealing potential stability problems over a similar range. To limit dissipation it is convenient to use a toneburst signal for these clipping tests.
The next two photos are oscilloscope traces showing examples of clipping behaviour:
The first of these is just a single notch when coming out of clipping, and this is typical of latch-up effects rather than instability. In this case it was caused by a bad choice of frequency compensation circuit such that the compensation capacitor charged up during clipping and had to discharge before normal linear operation could return. A change to the compensation arrangement was needed to cure this.
Stability problems generally have a different appearance of the type shown in the second photo. Here a short burst of oscillation occurs when coming out of clipping, but in this case the effect continues long after this as seen from a slight ripple on the trace. A change in the value of the compensation capacitor was needed to remove this effect. The positive and negative clipping look different, which is not uncommon, here the positive clipping appears to include a latch-up effect in addition to the stability problem.
Had I relied only on observations of square-wave ringing with a 2uF load below clipping I would have said there were no stability problems to worry about, and stopped there without doing the necessary modifications.
It is known that the choice of test signal rise-time can often have a great effect on observed 'ringing', and it is possible to claim 'excellent transient response' just by careful choice of the rise-time of the test signal. This was mentioned in one of the Douglas Self articles, "The Audio Power Interface", Electronics World Sept.1997 p717-722.
The low-pass filter used at the input of my own amplifiers helps give a smooth square wave output with little ringing, but it was not included for this purpose. Anyone who still wants to reduce ringing further in the mosfet amplifiers could try reducing the damping resistor in parallel with the inductor, maybe to one ohm.
Waveform and Spectrum Analysis
by Lloyd Butler VK5BR
The article is divided into two sections. Section A deals with typical CRO waveforms which might indicate certain characteristics or fault conditions in the electronic equipment being tested. The section shows various waveforms associated with square wave testing, sine wave testing, measurement of rise time and overshoot and measurement of phase shift. This section is part of the article "Measurement of Distortion" published in Amateur Radio, June 1989 (ref.1).
Section B displays Spectrum Analyser waveforms for sine wave, square wave, triangular wave and modulated signals. Also displayed are typical spectrograms made to measure frequency response or the characteristics of filters. This section was originally published in Amateur Radio, September 1987 (Ref 2). )
Section A
Waveforms using the Cathode Ray Oscilloscope (CRO)
SQUARE WAVE TESTING
One method of assessing frequency response (and sometimes other characteristics) is to feed a square wave to the input of the device under test and examine its output on a CRO. The square wave is made up of a fundamental frequency and all odd harmonies, theoretically to infinity. A deficiency within the frequency spectrum, from the fundamental upwards, will show a change in the shape of waveform. The test is subjective rather than precise but gives a good indication of the response.
Typical response patterns taken from a reference source are shown in figure 1. The captions under the patterns decribe the various operational conditions and the effect of loss of low or high frequency response is illustrated. Further patterns shown in figure 2 also illustrate the effect on the waveforms when relative phase delay is changed over part of the frequency spectrum. Also observe in figure 1(J) how the ringing from oscillation in the circuit under test is initiated by the steep edge of the square wave. This is a test result on how the circuit might handle a transient which might not have been detected in carrying out a sine wave frequency response check.
Related to frequency response, there is a specification called "transient response' which is the ability of a device to respond to a stop function. "Rise time" is one measure of transient response and is the time taken for the signal, initiated from a stop function, to rise from 10 percent to 90 percent of its stable maximum value. Another measure is the percentage of the stable maximum value that the signal over-shoots in responding to the step. Figure 3 shows how the square wave, in conjunction with a calibrated CRO, can be used to measure rise time and overshoot.
Rise time is also measure of the maximum slope of any sine wave component and hence is directly related to the limits in high frequency response. Together, rise time and overshoot define the ability of a device to reproduce transient type signals. Another specification commonly used in operational amplifiers is the 'slew rate" given in volts per microsecond. Such amplifiers have limitations in the rate of change that the output can follow and this is defined by the slew rate. The greater the output voltage, the greater is the rise time and hence the greater the output voltage, the lower is the effective bandwidth. Slew rate is equal to the output voltage step divided by the rise time as measured over the 10 percent to 90 percent points, discussed previously. It is an interesting observation that, in specifying frequency response, output voltage should also be part of the specification.
HARMONIC DISTORTION
Harmonic distortion in any signal transmission device results from non-linearity in the device transfer characteristic. Additional frequency components, harmonically related to frequencies fed into the input, appear at the output in addition to the reproduction of the original input components.
Measurement of harmonic distortion can be carried out by feeding a sine wave into the input of the device and separating the sine wave from its harmonics at the output. Distortion is measured as the ratio of harmonic level to the level of the fundamental frequency. This is usually expressed as a percentage but sometimes also expressed as a decibel. Distortion Meters and types of distortion are described in the original article (Ref 1). Intermodulation distortion is described in ref 3.
SINE WAVE TESTING
Subjective testing for harmonic distorton can be carried out by feeding a good sine wave signal into the device under test and examining the device output on a CRO. Quite low values of distortion can be detected in this way.
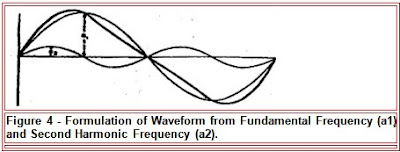
Some idea of the order of the harmonic can often be determined from the shape of the waveform. Figure 4 illustrates the formation of a composite waveform from a fundamental frequency and its second harmonic at one quarter of the fundamental Amplitude. Figure 5 illustrates similar formation from a fundamental frequency and its third harmonic, also a quarter of the fundamental amplitude. In Figure 5(b), the phase of the harmonic is shifted 180 degrees to that in Figure 5(a), and in Figure 5(c), the phase is shifted 90 degrees to that in (5a). The figures show that the composite wave forms can be quite different for different phase conditions making resolution sometimes tricky.
Some distorted waveforms directly indicate an out of adjustment or incorrect operating condition. The clipped waveform of Figure 6(a) shows the output of an amplifier driven to an overload or saturated condition. Figure 6(b) is clipped in one direction indicating an off-centre setting of an amplifier operating point. Figure 6(c) shows crossover distortion in a Class B amplifier.
Another method of testing, using sine waves, is to feed the monitored device input signal to the X plates input of the CRO and the device output signal to the Y plates input of the CRO. This plots the transfer characteristic of the device, that is, instantaneous output voltage as a function of instantaneous input voltage. X and Y gain is adjusted for equal vertical and horizontal scan. A perfect response is indicated by a diagonal line on the screen, or with phase shift, an ellipse or circle. Figure 10 shows various fault wave forms taken from one reference source. The different effects are explained in the diagram captions
The same connection can be used to measure phase shift between two sine wave signals of the same frequency such as measuring the phase shift between the output and input of an amplifier. Typical measurements are shown in Figure 8. A straight forward sloped diagonal line indicates no phase shift. A straight reverse sloped diagonal line indicates 180°. A circle indicates 90° and an elipse 45° or 135°.
If a dual trace CRO is available, the two signals can be displayed together, one on each vertical or Y trace with normal X sweep. In this case, it is simply a matter of scaling off the phase difference along the Y axis graticle.
Section B - Spectrum Analyser Waveforms
Over the years, the cathode ray oscilloscope (CRO) has been a universal instrument for examining analogue signals. Rapid advances in technology have led to a era of microcomputer controlled, digitally controlled test equipment, not the least of which is the modern spectrum analyser which enables greater precision analysis of analogue signals than is possible with the CRO. A spectrum analyser plots signal amplitude (or signal power) as a function of frequency compared to the CRO which plots signal amplitude as a function of time.
The spectrum analyser is not the type of equipment normally within the reach of the radio amateur and because of this, it was thought that it would be of interest to illustrate a few spectrum plots of well-known waveforms.
BASIC WAVEFORMS
Figure 1 shows the spectrum of a sine wave oscillator with fundamental at 1000 Hz and harmonics up to 20 kHz. The highest level harmonic at 7 kHz is 70 dB below the fundamental, representing a harmonic distortion of 0.03 percent. This is a very good oscillator which would not be matched by many laboratory instruments. It can also be seen that the noise floor is about 95 dB below the fundamental and this is also very good. The oscillator noise level might be even better than this as much of the noise is due to the spectrum analyser itself.
Figure 2 shows a 1000 Hz square wave. A perfect square wave generates odd harmonics to infinity with an amplitude 1/n relative to that of the fundamental or (20 log n) dB below the fundamental. ('n' is the order of harmonic). For n = 3, 5, 7 and 9 this calculates to -9.5, -14, -16.9 and -19.1 dB respectively, very close to the readings shown in Figure 2.
Figure 3 is the same square wave plotted out to 200 kHz and showing the apparently unlimited spread of harmonics. From this, it is easy to see why a low frequency square wave oscillator can be used as a marker generator over a wide frequency range.
Figure 4 shows a 1000 Hz triangular wave. A perfect triangular wave also generates odd harmonics to infinity, but each amplitude is (l/n) squared relative to the fundamental or (40 log n) dB below the fundamental. For n = 3, 5, 7, and 9, the calculation is -19, -28, -33.8, and -38.2 dB respectfully, again very close to the readings shown.
MODULATION
Figure 5 shows a 1 MHz carrier frequency, amplitude modulated by a frequency of 1 kHz to a modulation depth of 50 percent. For this case, the two side frequencies, 1 kHz either side of the carrier, are 12 dB below the carrier level, or a quarter of its amplitude. Other side frequencies at 2 kHz and 3 kHz, either side of the carrier, are the result of harmonics either in the original modulating tone or distortion caused by the modulation process. The 2 kHz side frequencies are about 30 dB below the 1 kHz side frequencies representing about three percent distortion in the system.
In Figure 6, the modulation level has been increased to 100 percent and the side frequencies, 1 kHz either side of the carrier, are now 6 dB below carrier level, or half its amplitude. The spectrum has been expanded to show many more harmonically related sideband components which now appear. Except for those close to the carrier, most of the components are more than 50 dB down and not of any great concern.
In Figure 7, the carrier is over-modulated and there is now a spread of sideband components about 30 dB down. If this were an amateur radio transmitter, other amateur stations in nearby suburbs would be complaining about sideband splatter.
Figure 8 Shows a 1 MHz carrier, frequency modulated by a 1 kHz tone with a deviation of 8.650 kHz, representing a modulation index of 8.650. It can be seen that there are many side frequencies all spaced by an amount equal to the modulating frequency (1 kHz). For this signal, a significant bandwidth of about 20 to 30 kHz is being utilised.
If we now examine Figure 9, which plots the amplitude of the carrier and side frequencies against the value of modulation index, we can see that there are a number of values of modulation index where the carrier level becomes zero. These are very convenient references to calibrate the amount of deviation. In Figure 8, the deviation has been set to produce the third carrier null at a modulation index of 8.650, so we know precisely that with our modulating frequency of 1000 Hz, our deviation is 8.650 x 1000 = 8850 Hz.
FREQUENCY RESPONSE
Another useful function of the spectrum analyser is to plot the frequency response of a four terminal device such as an amplifier or a filter. In this case, the analyser frequency sweep generator is fed to the input of the device and the output of the device is fed to the input of the analyser. Typical plots of a low pass filter and a bandpass filter are shown in Figures 10 and 11 respectively
Lenny Z Perez M
EES
Get news, entertainment and everything you care about at Live.com. Check it out!

















No hay comentarios:
Publicar un comentario